- Sketch the graph of \(F\) on the given domain.
- Find the average temperature, to the nearest degree Fahrenheit, between \(t = 6\) and \(t = 14\).
- An air conditioner cooled the house whenever the outside temperature was at or above 78 degrees Fahrenheit. For what values of \(t\) was the air conditioner cooling the house?
- The cost of cooling the house accumulates at the rate of $0.05 per hour for each degree the outside temperature exceeds 78 degrees Fahrenheit. What was the total cost, to the nearest cent, to cool the house for this 24-hour period?
Solution
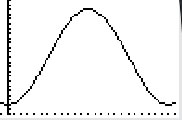
Solution
\(\frac{1}{14 - 6} \int_{6}^{14} (80 - 10\cos{ \left( \frac{ \pi t}{12} \right)) } \, dt = 87.162\). Answer: 87 degrees FSolution
\(80 - 10\cos{ \left( \frac{\pi t}{12} \right) } - 78 \geq 0 \implies 5.231 \leq t \leq 18.769\).Solution
Let \(p = 5.231\) and \(q = 18.769\text{. Then } 0.05 \int_{p}^{q}(80 - 10\cos{ \left( \frac{ \pi t}{12} \right)} - 78) \, dt = 5.096\); Answer: $5.10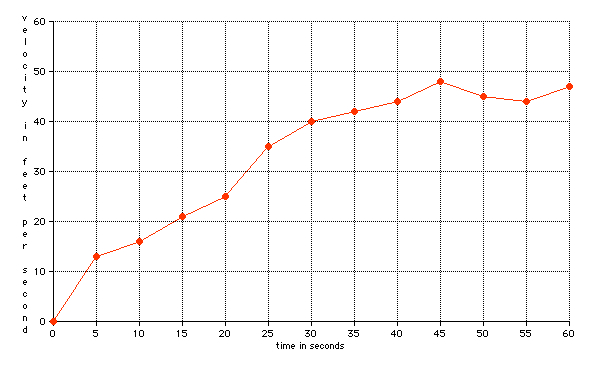
| \(t\) (seconds) | \(v(t)\) (feet per second) |
|---|---|
0 |
0 |
5 |
13 |
10 |
16 |
15 |
21 |
20 |
25 |
25 |
35 |
30 |
40 |
35 |
42 |
40 |
44 |
45 |
48 |
50 |
45 |
55 |
44 |
60 |
47 |
- During what intervals of time is the acceleration of the bike positive? Give a reason for your answer.
- Find the average acceleration of the bike, in feet per second squared, over the interval \(0 \leq t \leq 60\).
- Approximate the acceleration of the bike, in feet per second squared, at \(t = 30\). Show the computations you used to arrive at your answer.
- Approximate \(\int_0^{60} v(t) \, dt \) with a Riemann sum, using the midpoints of six subintervals of equal length. Using correct units, explain the meaning of this integral.
Solution
Acceleration is positive on (0, 45) and (55, 60) because the velocity is increasing on these intervals.Solution
Av. accel \( = \frac{47}{60} \frac{\text{ft}}{\text{sec}^2}\)Solution
\( \frac{v(35) - v(25)}{10} = \frac{7}{10} \frac{\text{ft}}{\text{sec}^2} \text{ or } \frac{v(35) - v(30)}{5} = \frac{2}{5} \frac{ \text{ft}}{\text{sec}^2} \text{ or } \frac{v(30) - v(25)}{5} = 1 \frac{\text{ft}}{\text{sec}^2} \)Solution
10(13 + 21 + 35 + 42 + 48 + 44) = 2030 ft. The particle moved 2030 feet to in th epositive direction from t = 0 to 60 seconds.- Use the trapezoidal rule with four equal subdivisions of the closed interval [0, 1] to approximate \(F(1)\).
- On what intervals is \(F\) increasing?
- If the average rate of change of \(F\) on the closed interval [1, 3] is \(k\), find \(\int_1^3 \sin{ \left( t^2 \right) } \, dt\) in terms of \(k\).
Solution
\( \frac{1}{2} \left( \sin{(0^2)} + 2\sin{\left( \frac{1}{4} \right) ^2} + 2\sin{\left( \frac{1}{2} \right) ^2} + 2\sin{\left( \frac{3}{4} \right) ^2} + \sin(1^2) \right) \frac{1}{4} \)Solution
\(F\) increases on [0, 1.772] and [2.506, 3] since \(\sin{\left( t^2 \right) } > 0\). (0, 1.772) and (2.506, 3]Solution
\(\frac{1}{3 - 1} \int_{1}^{3} \sin{ \left( t^2 \right) } \, dt = k\), so \( \int_{1}^{3} \sin{ \left( t^2 \right) } \, dt = 2k\)- Find \(g(3), g'(3)\) and \(g''(3)\).
- Find the average rate of change of \(g\) on the interval \(0 \leq x \leq 3\).
- For how many values \(c\), where \(0 < c < 3\), is \(g'(c)\) equal to the average rate found in part (b)? Explain your reasoning.
- Find the x-coordinate of each point of inflection on the graph of \(g\) on the interval \(0 < x < 7\). Justify your answer.